How does the Gerrymander Sequence Continue?
Manuel Kauers, Christoph Koutschan, and George Spahn
Abstract
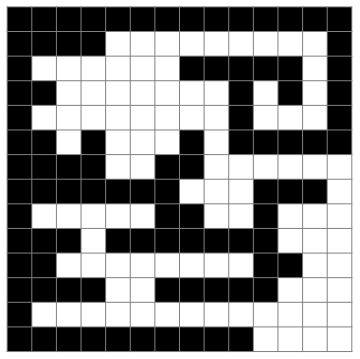
We compute a few additional terms of the gerrymander sequence (OEIS A348456) and provide guessed equations for the generating functions of some sequences in its context. Sequence A348456 counts the number of ways to dissect a 2n x 2n chessboard into two polyominoes each of area 2n2 (an example for n = 7 is shown above). Previously only the first three terms of this sequence were known. We find
A348456(4) = 7157114189
A348456(5) = 49852157614583644
A348456(6) = 28289358593043414725944353
A348456(7) = 1335056579423080371186456888543732162
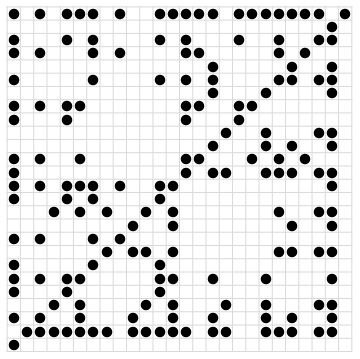
by employing the transfer matrix method. For example, for n = 2 we construct the following 26 x 26 matrix (non-zero matrix entries are illustrated by a bullet:
Paper
The material on this webpage accompanies the article How does the Gerrymander Sequence Continue? by Manuel Kauers, Christoph Koutschan, and George Spahn. This paper has been published in the Journal of Integer Sequences, vol. 25 (2022) Article 22.9.7. It is also available on arXiv:2209.01787.Supplementary electronic material
We provide a Mathematica notebook- Gerrymandering.nb (Mathematica notebook, last update 07.11.2022)
- Gerrymandering.cdf (Mathematica computable document)
- Gerrymandering.pdf (pdf printout of the notebook)
Here are Mathematica files containing the rational generating functions for slim rectangular boards of fixed width and varying length; x marks the width and z the number of black cells:
We also have the algebraic equation and the differential equation equation of the generating function for the sequence A167247.Follow-up work
In an impressive calculation and using a different approach based on the enumeration of self-avoiding polygons, Tony Guttmann and Iwan Jensen succeeded to obtain four more terms of the gerrymander sequence, i.e., A348456(8), ..., A348456(11). We highly recommend to read their excellent paper The gerrymander sequence, or A348456.Contact: